In Part 1 of this blog series, we illustrated how applying a prudent amount of leverage to a risk parity strategy allows investors to harvest a “diversification premium,” potentially leading to a more efficient outcome for investors who require a risk and return profile similar to global equity markets. The risk parity strategy accepts the underlying uncertainty of expected returns for financial assets and thus diversifies its portfolio “bets” across a wide variety of asset classes to harvest risk premia in a more reliable fashion.
In the second installment of this blog series on risk parity, we are going to dive into how dynamically adjusting the leverage applied to the strategy can result in increased stability of the portfolio’s return profile. Actively adjusting the amount of leverage applied to the portfolio is how the strategy scales the size of its bets based on changes in market risk.
We return to our analogy of Annie the poker player that we introduced in Part 1 and her experience in the casino on a nightly basis. One of the most popular types of poker is no-limit Hold’em. As the name states, there are no limits on betting, so we can now relax our assumption from Part 1 where the tables were either high or low stakes and did not vary throughout the night.
This example will show how dynamically adjusting leverage in a risk parity strategy is akin to adjusting your betting strategy at a poker table.
We imagine that Annie is playing at a table with five opponents and there is one more round of betting that will commence before the last community card is revealed. Annie has a flush draw but needs one more spade to complete her flush, which would give her a very high probability of winning the hand.

Now that the stage is set, we turn to how Annie’s betting patterns might change based on not only the probability of her hitting a spade, but also the varying uncertainties of her winning the pot should she in fact hit the flush.
At this point, Annie would only be willing to bet if there is positive expected value from her doing so – if her reward outweighs her risk. There is approximately a 20% chance of another spade being drawn from the deck. Annie should only be willing to bet or call if she is risking less for the pot than the odds of drawing an additional spade. If the total pot at stake is $500 but Annie needs to risk another $50 to call, then she should call. She is risking $50 for a 20% chance to win $500, yielding an expected value of $100!
Expected value and bet sizing are easy when all the information is provided and you can make a rational decision based on probabilities. The challenging aspect of poker is that you never have all the available information! This is not unlike financial markets – you may expect the Canadian equity market to return +7.0% on an annualized basis based on the last twenty years of historical data, but there is no guarantee this will occur.
Although Annie may have a 20% chance of hitting her flush, she doesn’t know what her opponents are holding. There is no guarantee that a flush will be good enough to ultimately win the hand. If there is only one other person in the hand, then there are only two cards which are unknown to Annie. In this situation, the range of potential hands that could beat Annie’s flush are narrower than if there are five other players all vying for the same pot. With five other players in the game, the number of hidden cards and the potential range of outcomes increases, prompting Annie to adjust her betting strategy to risk less since there are more possible outcomes where she loses. As the number of players in a hand increases, even if Annie hits the flush she is hoping for, there may be another player that has a better flush or a full house, both of which could beat Annie’s hand. With more players in the game, Annie’s uncertainty increases and thus dictates that she should bet more conservatively to control against realizing a “bad beat” that wipes out her bankroll.
As a poker hand progresses, the range of possible outcomes generally narrows. Early betting rounds with many players result in the most uncertainty and largest number of possible outcomes. Because of the large number of possibilities, we see smaller individual bets from players. In later betting rounds with fewer players, individual bet sizes become larger as the uncertainty reduces and we have a better understanding of what our risks are.
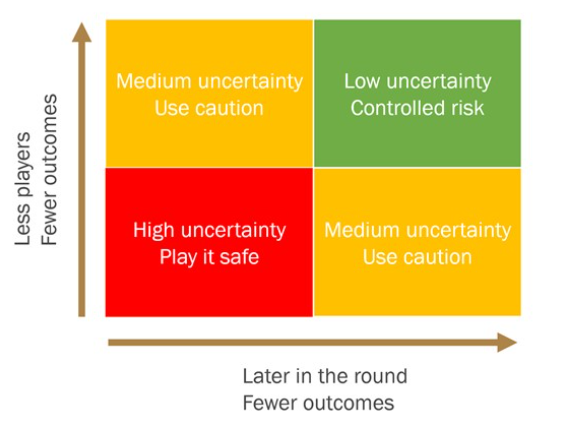
Annie is exposed to uncertainty based on the betting round and number of players remaining in a hand. In financial markets, market volatility exposes us to uncertainty of future outcomes. Just as Annie adjusts her betting strategy based on varying levels of uncertainty, we can adjust the leverage of a risk parity portfolio as market volatility changes. As volatility (risk) in financial markets increases, so does the range of potential outcomes. If volatility increases from 15% to 30%, that is the equivalent of doubling your bet size. Further, when volatility doubles, our return expectations don’t also double. In fact, high volatility typically occurs during periods of market stress when the risk of loss is high. We are being asked to take twice the risk for the same (or lower) reward! Most investors would balk at such a suggestion.
We also know that the mean and median of expected return paths diverge when volatility rises, which inherently lowers the probability of realizing your investment targets. It therefore makes strategic sense to lower your leverage (bet size) when volatility is high (there are more players in a hand) even though your expected asset class return (value of winning the hand) based on known information stays the same.
We previously viewed the multi-asset risk parity strategy through the lens of using a static leverage ratio to target the volatility of global equities. The result is a more efficient portfolio with a higher return than equities for the same risk level. When faced with a high required return target, most investors will increase their exposure to equities in hopes of generating that higher return, but concentration risk can be disastrous to terminal portfolio values as the probability of ruin increases.
Using a risk parity strategy that utilizes a judicious amount of leverage reduces the need to rely on concentrated bets required to meet a target return objective. Instead of going all-in on a single hand at the poker table, we can simply play the long game and raise the bet over multiple hands to accomplish the same goal.
While risk parity with a static leverage ratio is a good start, we can take it a step further by dynamically adjusting leverage to help ensure we keep our bet sizes consistent with how the market is behaving. To apply dynamic leverage, first we create a predictive model to forecast future volatility. It is a stylized fact accepted by both academics and practitioners that volatility tends to cluster and mean revert. We use statistical models which exploit this information to create expectations of future volatility and adjust our leverage based on these predictions. By continually adjusting our leverage, we are able to keep our bet sizes consistent over time.
Using a consistent bet size stabilizes our return streams. We see smaller drawdowns by lowering our bet size during high volatility and more efficient capitalization by raising leverage during low volatility bull markets. These two forces work together to allow dynamically leveraged risk parity models to harvest the diversification premia and generate alpha.
We can see this effect at work by constructing 250 alternative risk parity portfolios and applying dynamic leverage rules. The rules for construction are:
The results are given in the figure below, where each portfolio is plotted once using static leverage and once using dynamic leverage. Visually, it is simple to tell that the dynamically levered gold lines seem to outperform. We confirm our visual hunch by plotting a histogram of the realized Sharpe ratios (return per unit of risk). Since the dynamic leverage model is more efficient than static leverage, we see the distribution of Sharpe ratios shifted to the right. This means that we are more likely to have a better Sharpe ratio with the dynamic leverage model.
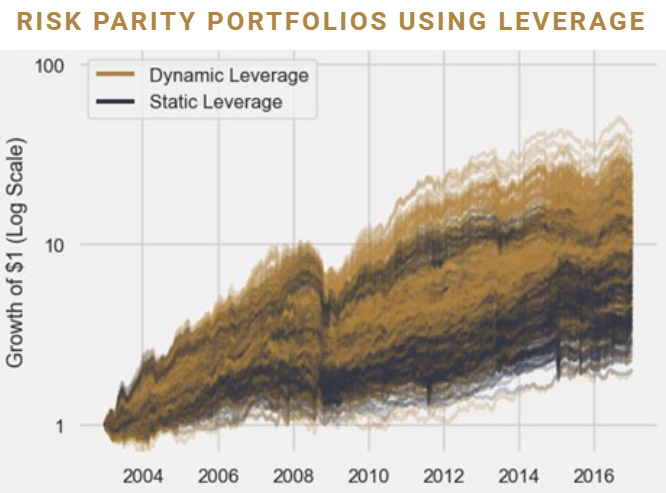
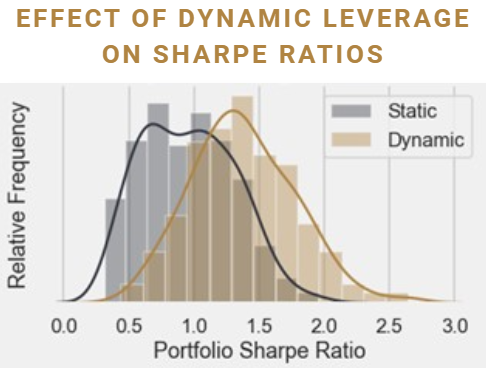
Intuitively, what is happening in the dynamically levered portfolios is that when the number of potential outcomes increases (due to high volatility), we reduce the amount of leverage employed and decrease our bet sizing until the volatility and potential outcomes stabilize. Sure, we’ll miss some big gains by reducing our market exposure, but we’re far more likely to stay alive by folding when markets go crazy. Those who stay at the table the longest, win the biggest.
The average compound annual growth rate across the simulations is 18% before all costs and fees. What if we wanted to achieve that level of return using pure equity? We can create an experiment to understand the implications of taking such a concentrated bet. Canadian equity has returned 7% per year on average. In order to achieve the average levered risk parity return of 18%, we need to apply about 2.5 times leverage to Canadian equity in order to replicate those results with a concentrated equity bet.
To understand what that looks like, we run a Monte Carlo simulation using bootstrapped Canadian equity return data between 2000 to 2020. Intuitively, we create different possible historical paths where we sample “chunks” of data (with replacement) and each “chunk” averages about a month’s worth of data. We generate 100 portfolios of concentrated Canadian equity and compare what sorts of return outcomes we realize over 20 years.
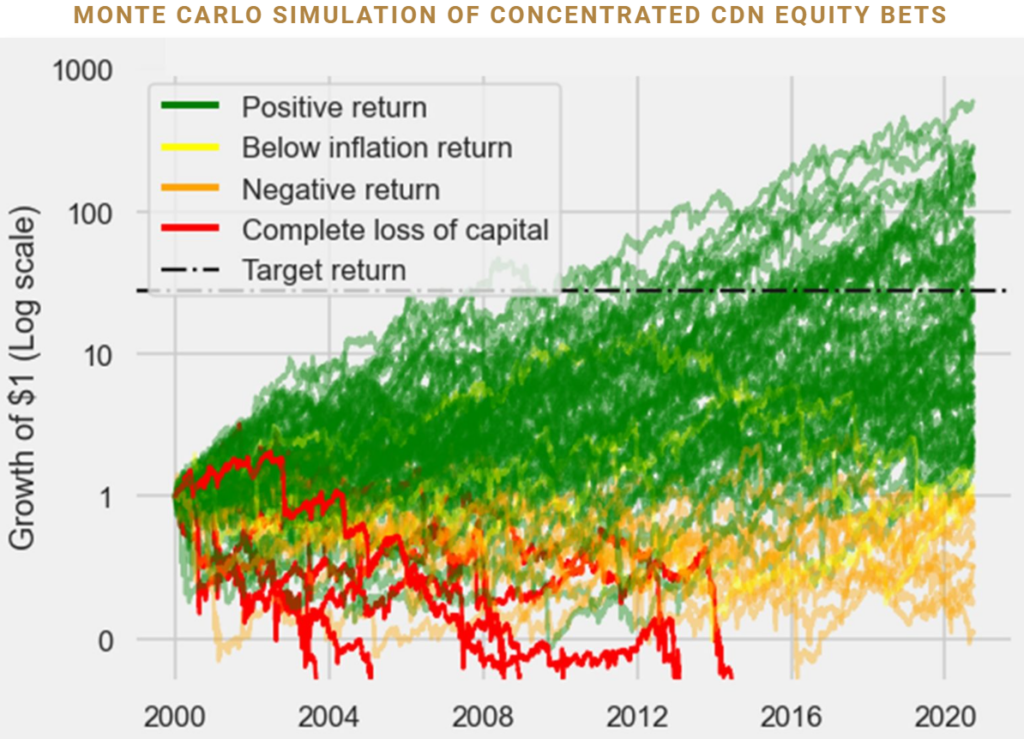
Clearly, in order to achieve the same return targets with a concentrated bet, we must take significantly more downside risk. Most investors would not be willing to risk a 5% chance of losing everything. In fact, 25% of the time your return is below inflation over 20 years!
For an investor to replicate the returns of a dynamically levered risk parity portfolio with equity markets, they must submit themselves to what many would consider unacceptable risk levels. Sure, some gamblers will win big, throwing caution to the wind and going all-in. However, we prefer to play the slow game, raising when the cards are in our favour and folding when they’re not.
If you’re curious, you are probably thinking, “Why can’t we apply dynamic leverage to a pure equity strategy? Wouldn’t that give us the best of both worlds – high expected returns with stable bet sizing?”
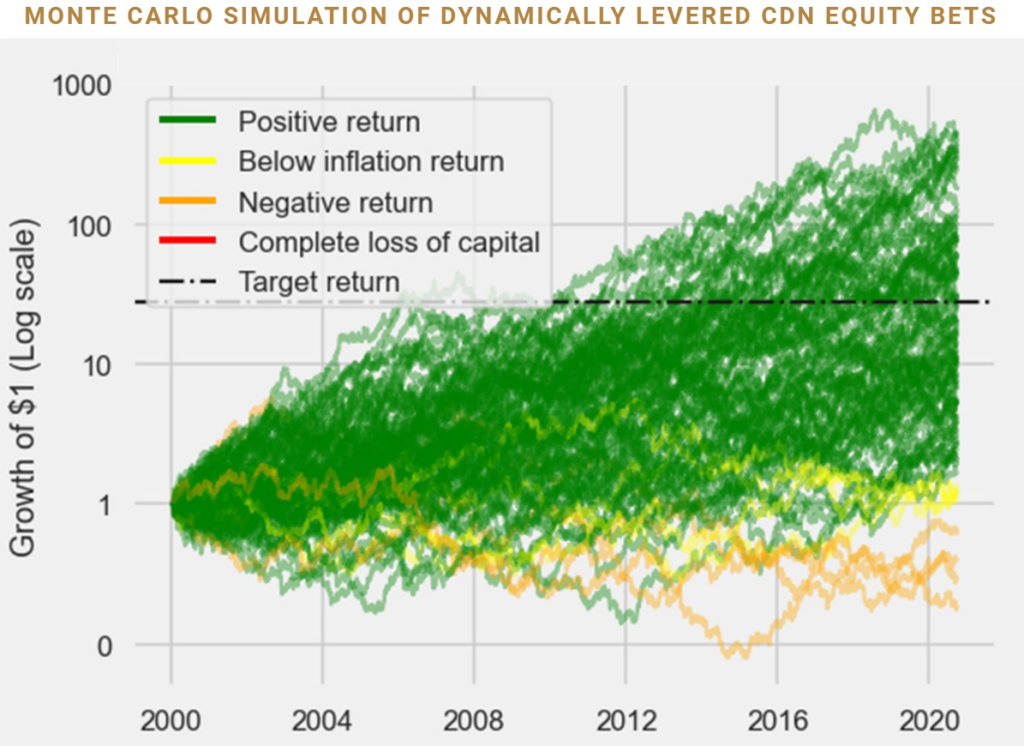
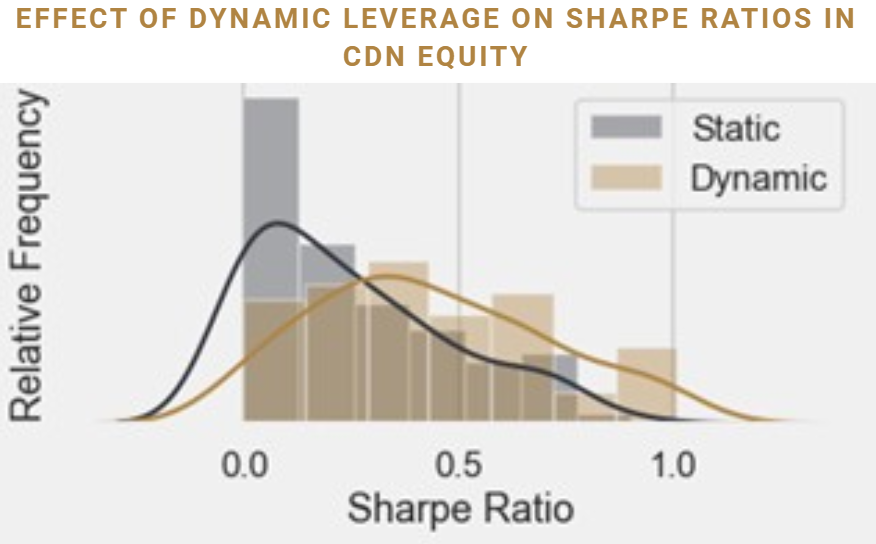
That looks better than the statically levered version – we’ve eliminated the risk of complete ruin, but we still have several cases of negative returns/low returns and only a third of the portfolios reach our investment targets. When we plot the distributions of Sharpe ratios, we see that the dynamically levered version is still better, but the effect is largely diminished compared to what we saw with the risk parity portfolios. Finally. So, what gives?
This is where the diversification premia we discussed in our last post comes in. Exploiting the diversification premium allows us to create a more efficient portfolio by mitigating the so-called “systematic risk” from the portfolio. Dynamic leverage allows us to reduce our exposure to unsystematic risk (market shocks, bear markets, etc.) when markets are in turmoil. Each technique is appreciable in its own right. By employing both, you get synergistic effects that make the sum greater than the parts.
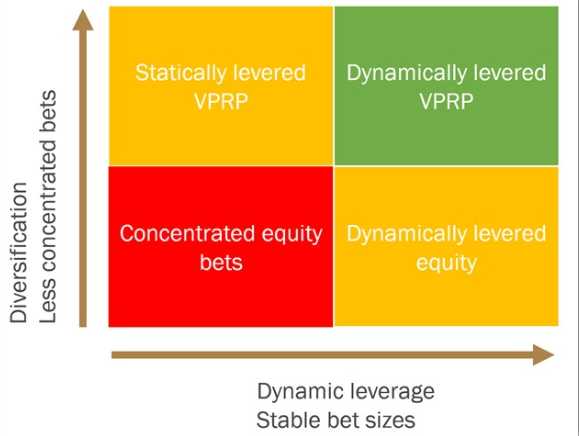
Through this two-part series on risk parity strategies, we have shown that investors don’t necessarily have to make concentrated bets on certain asset classes in order to achieve desired return targets. Instead, investors can construct a diversified portfolio of asset classes to create a more efficient strategy and utilize a prudent amount of leverage to target a desired risk profile. Furthermore, by dynamically adjusting leverage, investors can shift the distribution of simulated return paths further to the right, allowing them a better chance at realizing a higher Sharpe ratio versus a similar strategy with static leverage. In an environment where both equities and nominal bonds are expected to produce lower than historical long-term returns due to lofty valuations, investors may want to consider assessing how a risk parity strategy can complement traditional investment portfolios.
DISCLAIMER:
This blog and its contents are for informational purposes only. Information relating to investment approaches or individual investments should not be construed as advice or endorsement. Any views expressed in this blog were prepared based upon the information available at the time and are subject to change. All information is subject to possible correction. In no event shall Viewpoint Investment Partners Corporation be liable for any damages arising out of, or in any way connected with, the use or inability to use this blog appropriately.


